This article will focus on the gravity drive.
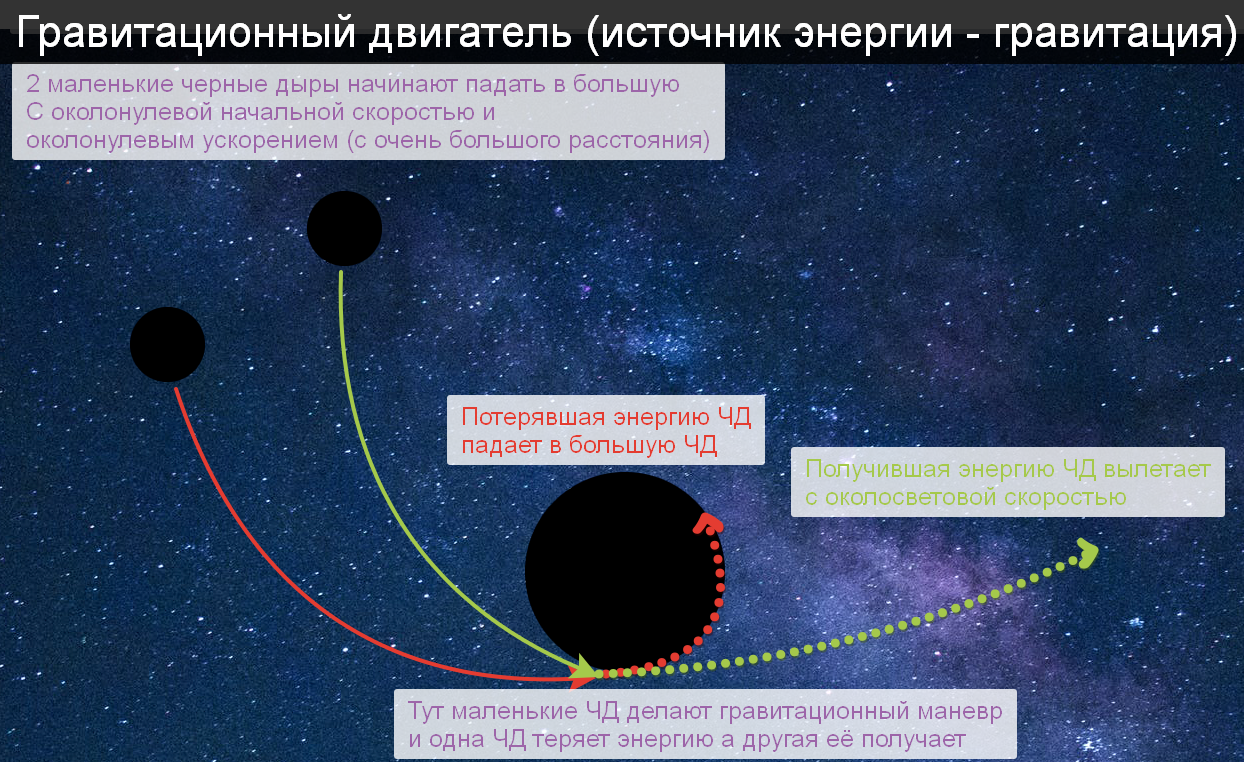
The picture shows the simplest scheme:
2 small black holes from afar begin to fall into a large central one.
Initially, no one has any energy - everyone starts with near-zero speed.
For example, let's take the solar system and squeeze the sun into a black hole.
We take the mass of small BHs thousands of times less than the Sun's - the mass matters, but for simplicity we take the same and insignificant relative to the central BH.
We take the initial velocity of small BHs to be the second cosmic one for the initial height.For example, if we start falling from the height of the Earth, then the initial velocity is ~ 42 km / s, if we start from the height of Jupiter, then ~ 18.3 km / s - i.e., the further , the lower the initial energy, it can be 100 m / s and near-zero, but for convenience we will start with the Earth's height and a velocity of ~ 42 km / s.
So: To begin with, let's throw one BH into the normal Sun at a speed of ~ 42 km / s along a parabolic trajectory - in this case, when approaching the Sun, it accelerates and near the "surface" of the Sun (695,700 kilometers from the center of the Sun itself) will accelerate to a speed of ~ 617 km / s, will fly close to the "surface" and begin to move away / decelerate, and at the height of the Earth its speed will be the same as initially 42 km / s.
Now we throw 2 BHs into the normal Sun approximately as in the first picture. There are a lot of options for initial parameters and you can throw with different initial speeds or different distances, but we will take the simplest and most visual, the same speed, the same distance to the Sun, but throw from different starting points.
So, initially small BHs are not connected with each other (the distance between them is millions of kilometers).
They start each with a speed of 42 km / s, and with the maximum approach to the Sun, their speed will be ~ 617 km / s, and at the same moment they will approach as close as possible to each other and make a gravitational maneuver to redistribute energy.
Briefly about gravity assist maneuvers from Wikipedia.


For 1 gravity assist, you can transfer a maximum of 50% of the energy, that is, it will not be possible to take all the energy from the "discharged" BH at once, and in this case the efficiency is 50%, but there are a lot of parameters and without going into details, the efficiency is 50% - it is very easy, 99% is already more difficult, but nevertheless it is also possible.
First, let's figure out how much energy can be obtained in this way (the efficiency is, as it were, 100%).
So, when approaching the Sun, we have 2 BHs at a speed of 617 km / s = energy of ~ 190 gigajoules per kilogram each. We take energy from one and give it to the other.
Total BH without energy will, as it were, “lie” on the “surface” of the Sun at zero speed.
A BH that has received energy will begin to move away from the Sun at a speed of 872 km / s, and when it flies off to the height of the Earth - at which it was originally, its speed will be ~ 618.5 km / s = energy 191 263 MJ per kilogram. And this is the energy that can be obtained by burning 4.3 TONS of gasoline !!!
Now we simply squeeze the Sun 100 thousand times (nothing is easier - than squeezing a thermonuclear bomb in the active phase) to a diameter of ~ 7 km - this is not yet a BH, but a neutron star. So we have the same mass - Solar, and even the matter itself is the same and nothing inside has changed, only the density has changed, but now we can get much more energy and if earlier we could approach the center of mass at a distance of 696 thousand kilometers and would collide with the "Surface" of the Sun, then now the "path is free" and we can fly hundreds of thousands of kilometers more receiving energy / acceleration.
How much energy can you get around a neutron star?
Near a neutron star, the speed will be hundreds of thousands of km / s (tens of percent of the speed of light) and there it is already necessary to take into account the effects of the Theory of Relativity, and unfortunately I am not a "gravitologist" and I cannot count the curves of space, but in one of the lectures of Sergei Popov, he said that when falling on a neutron star, the energy is 20% of Those. Near a neutron star, from each dropped kilogram, we can receive 20% of the energy that we would receive from the annihilation of 1 kg of matter and antimatter. And this is from a neutron star, if we squeeze it to a black hole, then the energy will be more than 20% of ...
How much energy can you get from gravity?
And this is the most interesting and difficult question. Of course, I'm not an astrophysicist, but I understand something in physics and mathematics, but I spent 300 hours on this question and in the end I had much more questions than I had initially. In general, further I will just tell you my thoughts on this matter.
So, we compress the Sun into a black hole and get a crystal clear gravitational engine. There are no thermodynamics, entropy, photons and electrons here = Electromagnetic interaction is not involved here at all !!! -Only mass and gravity, and at the same time we can get energy. In a system of 3 bodies, where initially there is no energy and everything does not move anywhere (almost), we can get a mass flying with a speed of near light. Those. In fact, in a 3-body system, gravity can repel, and potential energy is not negative energy - it can be made positive.
At the density of a neutron star, you can get 20% of the energy from , BH has a higher density and more energy. The minimum flight distance near the star is limited by the surface of the Star itself, the BH has no surface, but there is an event horizon from under which nothing can be pulled out and energy can be taken only above the horizon. And the event horizon just begins where the second cosmic velocity becomes equal to ... And in fact, the maximum energy that can be taken from the discarded body is equal to the energy of annihilation. And we, as it were, can get rid of the mass by annihilating matter with antimatter and get energy, or we can throw the mass into a black hole (in fact, nowhere) and get exactly the same energy and in the same way lose mass forever and irrevocably.
But at the same time - Black holes are much more "real" than antimatter, it is generally impossible to find pure antimatter in the universe, and black holes are simply lying around in a vacuum. In the galaxy, there are double black holes orbiting each other at a near-light speed, formed naturally.

BH slows down the stone is accelerating. If, for example, we again take the solar system (in the center there are 2 rotating BHs with a mass of 0.5 solar each), then we drop 2 stones from the Earth, one stone remains in the center - after the braking gravity maneuver, it will remain in the orbit of the center of mass - around which they themselves rotate BH and will eventually fall into one of the BH, and the second accelerated stone will arrive at the Earth at a near-light speed. A rock flying at near-light speed is great, but we need electricity. The dumbest way to get electricity is to push this stone with the Moon, that is, the stones fly into the Moon at near-light speed, it heats up and starts to glow like a light bulb - we get electricity from the light. Colliding at this speedthe power of the explosion will be ~ 2 million times greater than the power of the bomb dropped on Hirashima (with the mass of the stone equal to the mass of the bomb). In fact, there are a lot of ways to get electricity using gravity, but for now, let's deal with the energy source itself.
We will have to spend the mass in the case of finding 2 Black holes only so that the black holes do not merge and are always at the same distance from each other. If you do not "waste" mass, but throw stones only to accelerate, then BHs will begin to converge
and someday merge, and to obtain energy, two are required - Rather, the main structural element is one dense body with high speed in orbit of a massive body, (the ideal is just a black hole moving at near-light speed around another black hole). And if they merge, then we will have only one BH, and with zero speed, so we need to keep a balance. But still the question arises: - How much energy can be taken before 2 BHs merge? - 100% off , if we have 2 BHs of 0.5 Sun Masses, then they will merge when we take energy equal to the annihilation of the whole Sun (depends on the initial distance between them, but even if initially they rotate in the orbit of Mercury ~ 120 million km from each other , then the total energy will be more than 99% of ). In fact, the Sun in 5 billion years of its existence will not produce so much energy, and in its entire life the Sun will "dissolve" less than 0.1% of its mass.
Thermonuclear fusion is generally bullshit compared to how much energy can be obtained using gravity. By the way, the BH mass does not affect the efficiency at all = the velocity near the event horizon is always the same. And in order to obtain energy, in fact, we do not need to have black holes of a huge mass, in fact, the mass of a BH can be even 1 kg and we can compress 2 watermelons to black holes, and everything will work the same way as with large black holes. Moreover, it is much easier to control small BHs than thermonuclear fusion (there is even an opinion that it is generally impossible to keep matter at a temperature of several billion degrees), and black holes can be kept with the same gravitational maneuvers. Therefore, a small and even portable reactor weighing several kilograms is quite possible. True, the smaller the NP, the more "angrier" it is,near the event horizon, huge tidal forces will tear apart any matter and you will get 2 small vicious and uncontrollable quasars. In general, dumping matter into small BHs is a bad idea.
Can light be thrown into Black Holes?
I'm certainly not an expert on curved spaces, but it seems like gravity assist should work with light as well. At least, I heard about such an experiment: At the institute they shone a "flashlight" vertically up / down. And photons going up lost energy (turned red), and photons falling down received energy (chenille). Those. Gravity transfers energy to light, which means that gravitational maneuvers with light should also work (the light passing behind the BH movement will “accelerate” / turn blue / receive energy, and the BH passing before the movement will “slow down” / redden / lose energy). Light has an impulse and can "push" the sail, so gravity assist should most likely work. Then the energy of gravity can be easily obtained even from very small black holes.
What about Hawking radiation?
Hawking radiation allows us to create a perpetual motion machine, we throw off mass and get energy, and it goes out again, we again throw it off and get energy, and it goes out again. Therefore, for all questions, contact Hawking himself, and even better to those who gave him the Nobel Prize. In my engine, no quantum numbers are violated - Everything is completely legal !!! Schrödinger's cat will be happy !!!
Although there is still a smell of perpetual motion machine here. The light pulse is
The higher the frequency, the greater the impulse, but the "braking" gravity assist will decrease the frequency, and in the extreme case, if you take all the energy from the light near the event horizon, then it will fall into a BH with zero energy, then how much will the BH mass increase?
Matter, by the way, has the same problem, if you take all the energy from a kilogram stone before dumping, the mass of the BH should increase by 1 kg. But the second kilogram stone that will carry away energy - when it collides with the Moon at near-light speed, fragments from it will fly out by 2 kg. ( works both ways). And as a result, we will again have 2 kg., And even the mass of the BH will increase by 1 kg. In total, the total mass of the universe will increase by 1 kg. And it seems that this problem is not being solved at all - the total mass of the universe will increase at any efficiency.
Or in some miraculous way, when dropped into a black hole with a speed / energy less than - the mass of the BH will decrease and then it turns out that with the help of gravity we can extract energy from the BH.
Either the mass of the universe will still increase ... but some kind of game will happen. Nevertheless, we will not be able to infinitely increase the mass, if we do all this conditionally in the orbit of the Earth, then over time, the event horizon will "reach" the Earth, and we will simply find ourselves in a black hole (we will not receive energy in this case). And then we will not be able to infinitely receive mass / energy. But all these problems will begin after the central mass has increased by a factor of millions.
In general, what kind of energy is this and where does it come from is a very interesting question and it is possible that this energy has nothing to do with - this is some other energy and there is much more of it. If you go from the other side and ask the question - why did the entire mass of the universe not collapse into a huge black hole? - She was pushed by some kind of energy ...
PS
In general, Black holes and turbid energy are all wonderful, of course, but this is an extremely distant future, and it is not clear how it will work with BH. In the next article, we'll talk about more realistic options. In fact, even in orbit around Jupiter, you can easily build a power plant out of mud sticks and foil - producing 900 MJ for every kilogram dropped, which is the energy of 25 liters of gasoline. In the next article I will try to estimate how much such an orbital structure will weigh.
PPS
I would also like to turn to the readers. Can someone translate the article into English? It's just that such articles take a lot of time, and in Russia no one really needs physics. At least demand is needed. And in general, where can you write such articles on English resources?
Sometimes, between states, I'm neither alive nor dead - I collapse on twitch .