
A diagram of a strong curvature of space-time near the event horizon of a black hole. The closer you get to a massive body, the more space is curved. As a result, you find yourself in a place from which even light cannot escape: inside the event horizon.
Most people, thinking about the Universe, imagine material objects located at huge cosmic distances from each other. Under the influence of its own gravity, matter collapses, forming such cosmic structures as galaxies. Gas clouds, collapsing, give rise to stars and planets. Stars emit light by burning fuel in nuclear fusion reactions. This light travels across the entire universe, illuminating everything that comes across. However, the universe is not only about the objects within it. There is also the fabric of space-time, which plays by its own rules - by the rules of the general theory of relativity (GTR). The fabric of space-time is curved in the presence of matter and energy, while the very curvature of the fabric of space-time dictates how matter and energy move. But what is, specifically, space-time is something "real",or just a calculating tool? The reader asks us about this:
What exactly is spacetime? Is it a real thing like atoms, or is it a mathematical construct used to describe how mass "generates" gravity?
Great question, and the topic is difficult enough to think about. Moreover, before Einstein's appearance, our understanding of the universe was very different from the current one. Let's go back to the distant past of the Universe, when we did not have the concept of space-time yet, and we will move forward until today.
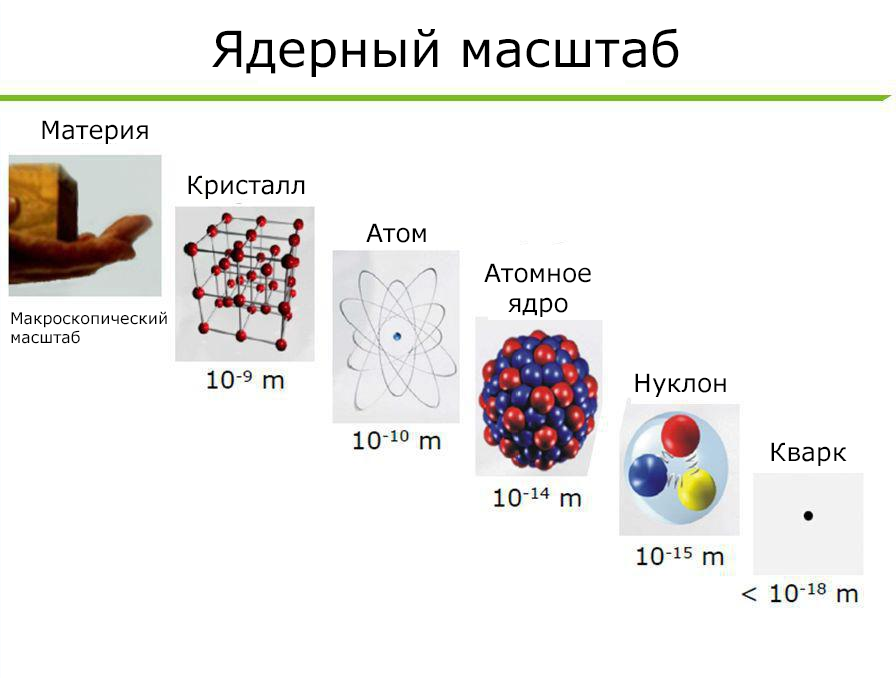
At all scales, from macroscopic to subatomic, the sizes of fundamental particles play little role in determining the final sizes of composite structures. Whether these building blocks of matter are truly fundamental point particles is still unknown. However, we understand the structure of the Universe from gigantic, cosmic scales to tiny, subatomic ones. For example, the human body contains about 1028 atoms.
At a fundamental level, we have long suspected that if you take any object in the Universe and start dividing it into smaller and smaller component parts, you can eventually achieve something indivisible. The word "atom" literally means "indivisible," from the Greek ἄτομος. The first mention of this idea occurs 2400 years ago, in Democritus... However, it is likely that the idea could have been invented earlier. Such indivisible entities actually exist - they are known as quantum particles. Despite the fact that we have called the elements of the periodic table atoms, subatomic particles - quarks, gluons and electrons (as well as those particles that do not occur in atoms at all) are truly indivisible.
All these quanta are bound together and make up all the constituent structures of the Universe we know today - from protons and atoms to molecules and people. And all these quanta, regardless of their type - matter or antimatter, whether they have mass or not, fundamental or composite, subatomic or cosmic - exist within the same Universe as we do.

If you know all the rules governing the movement of an object in space-time, as well as the initial conditions and all the forces acting between the object and the rest of the system, you can predict how it will move through space and time. But the location of an object cannot be specified precisely without adding a temporal one to the spatial coordinates.
And this is important, because if you want all things in the Universe to do something with each other - interact, connect, form structures, transfer energy - you need to have a way to do this. Imagine a play in which all the characters are written, the actors are ready to play them, the costumes are prepared, all the lines are written and learned. The only thing missing is a very important thing - the scene.
What is the role of the scene in physics?
Before Einstein appeared, the stage was set up by Newton. All the "actors" of the Universe could be described by a set of coordinates - a location in three-dimensional space and a moment in time. It was like a Cartesian grid — a three-dimensional structure with x, y, and z axes, where each object can have an impulse that describes how it moves through space as a function of time. Time itself was considered linear, running at a constant speed. In Newton's view, space and time were absolute.

We often think of space as a three-dimensional lattice, although this is an oversimplification that depends on the frame of reference. In fact, space-time is curved in the presence of matter and energy, and the distances in it are not fixed, but change with the expansion or contraction of the universe.
However, the discovery of radioactivity at the end of the 19th century cast a shadow of doubt on Newton's picture of the world. Learning that atoms can emit subatomic particles moving at the speed of light, we realized something amazing: when a particle moves at a speed close to the speed of light, it perceives space and time in a completely different way from an object moving slowly or at rest.
Unstable particles, which decay very quickly at rest, lived the longer, the closer their speed was to the speed of light. These particles traveled longer distances than they should have, based on their speed and lifetime. And when trying to calculate the energy or momentum of a moving particle, different observers (moving at different speeds relative to it) received non-coinciding values.
It turns out that something was wrong with Newton's concept of space-time. At speeds close to the speed of light, time lengthens, distances contract, and energy and momentum depend on the frame of reference. That is, your perception of the universe depends on how you move.
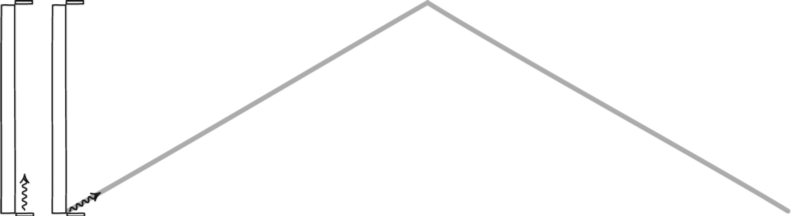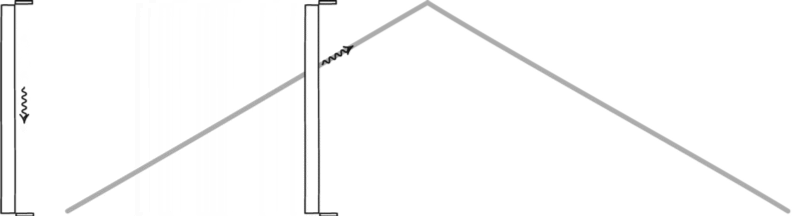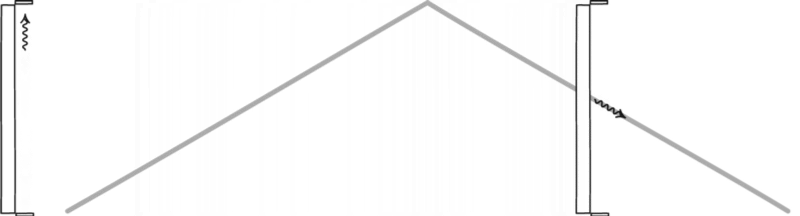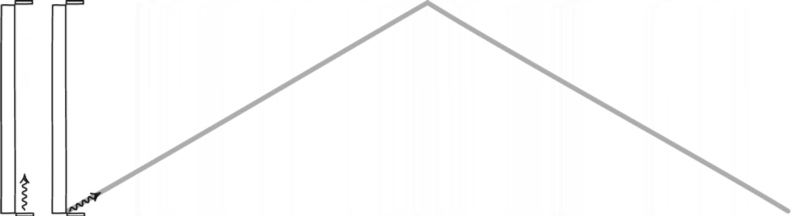
A light clock, in which a proton is reflected from two mirrors, can count the time for any observer. And although two observers may disagree about how much time has passed between the two moments, they can agree on the laws of physics and the constants of the universe, in particular, about the speed of light. For a stationary observer, time goes on as usual, and for a fast-moving person, the clock will go slower than for a stationary one.
Einstein is responsible for an outstanding breakthrough in the concept of reality, which described which quantities do not change as the observer moves, and which depend on the frame of reference. For example, the speed of light is the same for all observers, as is the rest mass of any amount of matter. But the distance between two points is highly dependent on your movement along the line connecting them. The speed at which your watch is running also depends on your movement.
Space and time turned out to be not absolute, as Newton thought, and were perceived by different observers in different ways. They turned out to be relative, which is why the theory is called the "theory of relativity". Moreover, there is a definite connection between the perception of space and time by a certain observer. A couple of years after Einstein's publication of the special theory of relativity (STR), it was derived by his former professor Hermann Minkowski. He deduced a single mathematical structure that includes space and time: space-time. As he himself wrote,
From now on, time itself and space in itself become an empty fiction, and only their unity retains a chance for reality.
Today this spacetime is still widely used, if gravity can be neglected: Minkowski space .

A cone of light, a three-dimensional surface made up of all possible rays of light coming and going from one point in space-time. The more you pass in space, the less you pass in time, and vice versa. Today, you can only be influenced by what was in the light cone of the past. In the future, you will only be able to perceive those things that are contained in your future light cone. This is an illustration of a flat Minkowski space, not a curved general relativity space.
But there is gravity in the real universe. This force does not act instantly across the vast expanses of space. It propagates at the same speed as all massless quanta: at the speed of light. All the rules formulated in SRT remain applicable, but to include gravity in the picture, something more was required: an idea of the presence of space-time of its own curvature, depending on the presence of matter and energy in it.
In a way, it's simple: if you place actors on the stage, the stage must be able to support their weight. If the actors are massive and the scene is not perfectly rough, it will deform in their presence.
The same phenomenon works with space-time: the presence of matter and energy bends it, and this curvature affects the distance (space) and the speed of the clock (time). Moreover, this influence is quite complex. If we calculate the influence of matter and energy on space-time, then the spatial and temporal effects turn out to be related. The lines of the three-dimensional lattice, which we represented in SRT, are curved in GRT.

The appearance of mass in an empty three-dimensional lattice causes its lines to bend in a certain way. They seem to be stretched towards the mass.
Space-time can be thought of as a purely computational tool, and stop there. In mathematics, even spacetime can be described with a metric tensor. This formalism allows you to calculate, like any field, line, arc, distance, etc. can exist in it in a certain, precisely described way. Space can be flat or arbitrarily curved, finite or infinite, open or closed, and consist of any number of dimensions. In general relativity, the metric tensor is four-dimensional (with three spatial and one time dimensions), and the curvature of space-time is determined by matter, energy and its internal tensions.
Simply put, the curvature of spacetime is determined by the contents of the universe. And then you can take the curvature of spacetime and predict how any piece of matter and energy will move and change over time. The rules of general relativity allow us to predict how matter, light, antimatter, neutrinos, and even gravitational waves will move through the universe. All of these predictions are in perfect agreement with our observations and measurements.

A signal from the GW190521 event associated with the appearance of gravitational waves, recorded by three detectors. The signal duration was about 13 ms, but it represents the energy equivalent to converting 8 solar masses into pure energy via Einstein's equation E = mc 2 .
What we don't measure is space-time itself. We can measure distances and time intervals - but these are all indirect sensing of the underlying spacetime. We can measure everything that interacts with us - bodies, instruments, detectors - however, interaction occurs only when there are two objects at one point in space-time, when an "event" is registered when they meet.
We can measure all the effects that curved spacetime has on the matter and energy of the universe, namely:
- Radiation redshift generated by the expansion of the Universe;
- Bending of light due to the presence of masses in the foreground;
- Entrainment of inertial reference frames in the presence of a rotating body;
- - , ;
- , , ;
And also many other influences. However, from the fact that we can only measure the effect of space-time on the matter and energy of the Universe, but not space-time itself, it follows that space-time behaves in a manner indistinguishable from a simple computation tool.
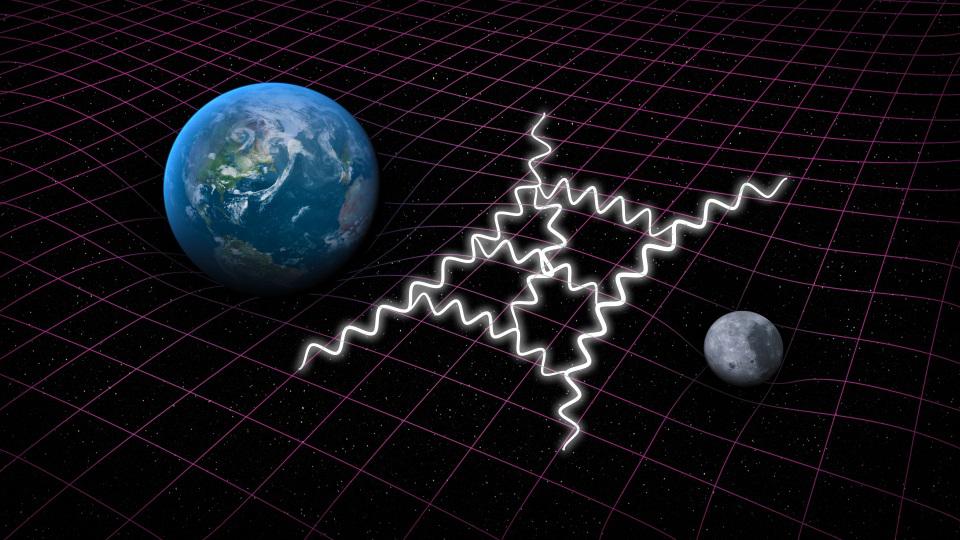
Quantum gravity tries to combine Einstein's general relativity with quantum mechanics. Quantum corrections for classical gravity are indicated in the form of loop diagrams, such as the one shown in the figure in white. If the Standard Model is expanded to include gravity, the symmetry describing the CPT (Lorentz symmetry) can only become approximate, and its violations can appear. However, so far no such violations have been observed in experiments.
But this does not mean that space-time is not a real physical entity. By observing the actors playing the play, you have the right to call the place where the play is going, a "stage", be it a field, a platform, bare ground, etc. Even if the play were performed in the weightlessness of space, you could simply note that a freely falling frame of reference is used as a scene.
In the physical Universe, as far as we know, the existence of objects and interaction between them is impossible without space-time. Where there is space-time, the laws of physics work, and there are fundamental quantum fields that underlie everything. In a sense, "nothing" is a vacuum of empty space-time, and talking about what happens in the absence of space-time makes no sense - at least from the point of view of physics. It makes no sense to talk about “where”, which lies outside the boundaries of space, and “when”, which is beyond the boundaries of time. Perhaps something like that exists, but we have no physical concepts of this entity.

Animation of interaction of space-time with mass moving through it. It can be seen from it that space-time is not just some kind of fabric. All three-dimensional space is curved in the presence of mass and energy. Several masses rotating around each other generate gravitational waves.
The most interesting thing is that we still have many unanswered questions about the nature of space-time. Are space and time quantum and discrete, divided into invisible regions, or are they continuous? Is gravity a quantum interaction, like all other known forces, or is it a classical, continuous web that stretches all the way to Planck scales?? If space-time differs from what general relativity tells us, then how exactly, and how can we detect it?
But, despite everything that space-time allows us to predict and know, it is not the same real entity as the atom. It is impossible to somehow directly "detect" space-time - you can only detect individual quanta of matter and energy that exist in your space-time. We described space-time in the form of Einstein's general relativity, and it successfully predicts and explains all the physical phenomena that we have ever discovered and measured. However, the question of what it is, and whether it is real or not, remains open for modern science.