David Hilbert's question about polynomials of the seventh degree, which was considered for a long time to be solved, opened a new network of mathematical connections for researchers
Success in mathematics is rare. Just ask Benson Farb .
“The problem with mathematics is that 90 percent of the time you fail, and you have to be the person who can accept it,” Farb once said over dinner with friends. When one of the guests, also a mathematician, was surprised that Farb was successful as much as 10% of the time, Farb admitted, "No, no, I greatly exaggerated my success rate."
Farb, a topologist at the University of Chicago, was happy to meet his latest setback - though, in all fairness, it's not entirely his credit. The question is connected with a problem, paradoxically solved and unresolved, open and closed.
The problem is the 13th of 23 mathematical problems that were not solved at the beginning of the 20th century. Then the German mathematician David Hilbert made this list , which, in his opinion, determined the future of mathematics. The problem is connected with solving polynomial equations of the seventh degree. A polynomial is a sequence of terms of an equation, each of which consists of a numerical coefficient and variables raised to a power; terms are connected with each other by addition and subtraction. The seventh degree means the largest exponent of all variables.
Mathematicians have already learned to skillfully and quickly solve equations of the second, third and, in some cases, fourth order. These formulas - including the familiar quadratic formula for the second degree - include algebraic operations, that is, arithmetic and root extraction. But the larger the exponent, the more confusing the equation, and it becomes more and more difficult to solve. Hilbert's 13th problem is the question of whether the solution to a seventh-order equation can be expressed in terms of a set of additions, subtractions, multiplications, divisions, and algebraic functions in at most two variables.

In 1900, David Gilbert compiled a list of 23 critical open problems.
Answer: probably not. For Farb, however, this is not just a matter of solving a complex algebraic equation. He said that Problem 13 is one of the most fundamental problems in mathematics, as it raises deep questions: How complex are polynomials, and how can they be measured? “A whole layer of modern mathematics has been invented to better understand the roots of polynomials,” Farb said.
This problem pulled him and the mathematician Jesse Wolfson of the University of California at Irvine down the mathematical rabbit hole, the moves of which they still study. She also brought in Mark Kissin , a Harvard number theorist and an old friend of Farb 's, to their excavation .
Farb acknowledged that they have not yet solved Hilbert's 13th problem, or even come close to solving it. However, they unearthed nearly extinct mathematical strategies, and explored the problem's links to various fields of knowledge, including complex analysis, topology, number theory, representation theory, and algebraic geometry. They applied their own approaches, in particular, combining polynomials with geometry and narrowing the range of possible answers to Hilbert's question. Also, their work proposes a way to classify polynomials by complexity metrics - an analogue of complexity classes related to the unsolved problem of equality of the classes P and NP .
“They were actually able to extract a more interesting version of interest from the interest,” compared to those studied previously, said Daniel Litt, a mathematician at the University of Georgia. "They show the mathematical community a lot of natural and interesting questions."
Opened, closed and reopened
Many mathematicians already thought the problem was solved. In the late 1950s, the brilliant Soviet scientist Vladimir Igorevich Arnold and his mentor Andrei Nikolaevich Kolmogorov published their proofs. For most mathematicians, Arnold-Kolmogorov's work closed this question. Even in Wikipedia - not the ultimate truth, but a rather reasonable intermediary in the search for knowledge - until recently the problem was marked as solved.

Vladimir Arnold and his mentor Andrei Kolmogorov in the 1950s proved one of the versions of Hilbert's 13th problem - but, perhaps, Hilbert was interested in another version of it.
However, five years ago, Farb stumbled upon a few intriguing lines in an essay by Arnold where the famed mathematician reflects on his work and career. Farb was surprised to learn that Arnold was describing problem 13 as open, and for forty years had been trying to solve a problem that he seemed to have already solved.
“There are scientific works where the thesis about the solution of the problem is simply repeated. They clearly don't understand the problem itself, ”Farb said. At the time, he was working with Wolfson, then a postdoc, on a topology project. When he shared the information he found in Arnold's work, Wolfson joined the project. In 2017, during a seminar dedicated to Farb's 50th birthday, Kissin heard Wolsfon's talk and was surprised to realize that their ideas about polynomials were related to issues in his work on number theory. He joined their team.
The reason for the confusion with this problem soon became clear: Kolmogorov and Arnold solved only one of its options. Their solution featured continuous functions — those that do not have sharp breaks or inflection points. These functions include familiar operations such as sine, cosine, exponential, as well as more exotic ones.
However, not all researchers agree that Hilbert was interested in them. "Many mathematicians believe that Hilbert was referring to algebraic functions, not continuous functions," said Zinovy Reichstein , a mathematician at the University of British Columbia. Farb and Wolfson are working on a problem that they believe Hilbert wanted to study.
Farb said problem 13 is a kaleidoscope. “You uncover this thing, and the more you study, the more directions and ideas it opens up,” he said. “It opens the door to a whole array of problems, reveals the entire beautiful web of mathematics.”
The roots of the problem
Mathematicians have been playing with polynomials since the invention of mathematics itself. Stone tablets 3,000 years old show how Babylonian mathematicians used the formula to solve second-order polynomials. It was the cuneiform predecessor of the very quadratic formula that is taught today in mathematics lessons. Formula shows how to find the roots of a polynomial - that is, the x values at which the expression ax 2 + bx + c, a polynomial of degree two, becomes zero.
Over time, mathematicians naturally became interested in the question of whether there are such clear and clear formulas for polynomials of higher orders. “The millennia-long history of this problem is to arrive at something just as powerful, simple, and effective,” Wolfson said.
The higher the degree of the polynomial, the more cumbersome they become. In the 1545 book Ars Magna [Great Art], the Italian polymath Gerolamo Cardano published formulas for finding the roots of third and fourth degree polynomials.
The roots of the cubic polynomial ax 3 + bx 2 + cx + d = 0 can be found using the following formula: The
formula for the fourth degree polynomial looks even worse.
“As the degree grows, so does the complexity, and the mountain of complexity looms,” said Kurt McMullen of Harvard. "How can we conquer this mountain?"
Italian mathematician Paolo Ruffini in 1799 argued that polynomials of 5th and greater degrees cannot be solved using arithmetic operations and extraction of roots. In 1824, the Norwegian mathematician Niels Henrik Abel proved this. ... In other words, there is no such formula for a polynomial of the fifth degree. Fortunately, other ideas have emerged that suggest ways to study polynomials of higher degrees that can be simplified through substitution. For example, in 1786 the Swedish lawyer Erland Bring showed that any equation of the form ax 5 + bx 4 + cx 3 + dx 2 + ex + f = 0 can be rewritten as px 5 + qx + 1 = 0, where p and q - complex numbers, the value of which is determined by a, b, c, d, e and f. This fact opened up new approaches to the hidden properties of polynomials.
In the 19th century, William Rowan Hamiltoncontinued the work of Bring and others. Among other things, he showed that to find the roots of a polynomial of the sixth degree, you need only the usual arithmetic operations, square and cubic roots, and an algebraic formula depending on only two variables.
In 1975, the American algebraist Richard Brower of Harvard introduced the idea of a "resolvent degree", which describes the minimum number of terms required to describe a polynomial of some degree. Less than a year later, Arnold and Japanese number theorist Goro Shimura, in another paper, introduced much the same definition.
In Brouwer's model, the first attempt at systematizing the rules for such substitutions, Hilbert's 13th problem is whether it is possible for polynomials of the seventh degree to have a resolvent degree less than 3. Later, he put forward similar conjectures about polynomials of sixth and eighth degrees.
However, all these questions are based on a more general one: what is the smallest number of parameters required to find the roots of any polynomial? What is the lower limit you can go?
Visual thinking
A natural approach to this question is to imagine what polynomials look like. The polynomial can be written as a function - for example, f (x) = x 2 −3x + 1, - and plot it. Then the search for roots is reduced to the fact that the function becomes equal to zero where its curve intersects the x-axis.
The higher the degree of the polynomial, the more complex its graph. Third-order functions in three variables produce smooth but twisted surfaces in three dimensions. By knowing where to look on these surfaces, mathematicians can learn a lot about the underlying polynomial structure.
As a result, attempts to understand polynomials involve many techniques from algebraic geometry and topology - branches of mathematics that focus on what happens to shapes when they deform, shrink, stretch, or otherwise change without discontinuity. “Henri Poincaré essentially invented topology, and clearly said he did it to understand algebraic functions,” Farb said. "At the time, people were having a hard time studying these fundamental connections."
Hilbert himself revealed a particularly interesting connection by applying geometry to this problem. By the time he drew up his list of problems in 1900, mathematicians already had a lot of tricks to lower the degrees of polynomials, but they still couldn't get any further. However, in 1927, Hilbert described a new trick. He began by identifying all possible ways to simplify ninth-degree polynomials, and found among them a family of special cubic surfaces.
Hilbert already knew that on every smooth cubic surface - an intricate figure described by a polynomial of the third degree - there are exactly 27 lines, no matter how twisted it looks. These straight lines shift as the coefficients of the polynomials change. He realized that knowing the position of one of them, he can simplify the ninth degree polynomial and find its roots. The formula required only four parameters - in modern terms, this meant that the degree of the resolvent did not exceed 4.
"Hilbert's amazing insight was that this miracle of geometry, originating from a completely different world, could be used to reduce the degree of the resolvent to 4", Farb said.
Moving towards a web of connections
When Kissin helped Farb and Wolfson understand the problem, they realized that the generally accepted view that Hilbert's 13th problem was solved had killed all interest in the geometric approach to the degree of the resolvent. In January 2020, Wolfson published a paper that revitalized this approach. She extended Hilbert's geometric inversion of ninth degree polynomials to a more general theory.
Hilbert concentrated on cubic surfaces to find solutions to ninth-degree polynomials containing only one variable. But what about higher degree polynomials? To solve this problem in a similar way, Wolfson thought, one could replace the cubic surface with some kind of “hypersurface” of higher order formed by these higher-order polynomials in many variables. The geometry of such surfaces is not well understood, but over the past few decades, mathematicians have proven that in some cases you can always find straight lines on them.
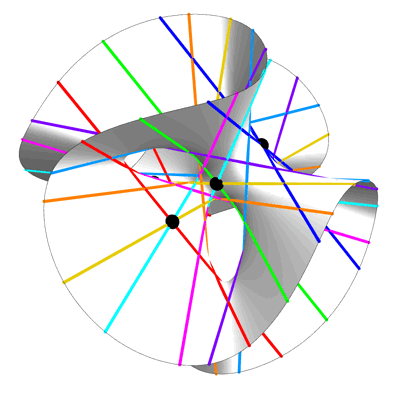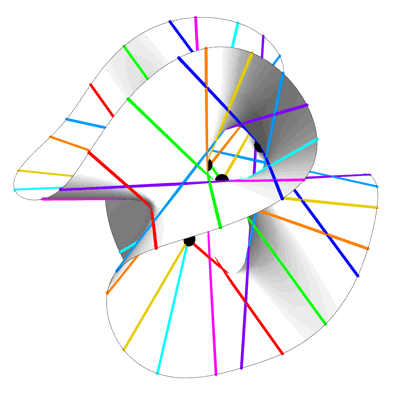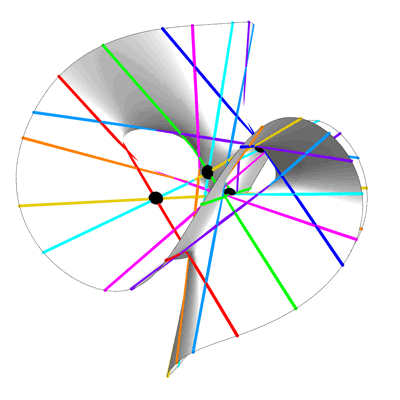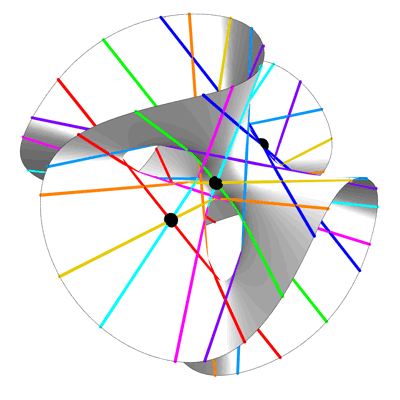
, , , 27 . . , «» .
Hilbert's idea of using straight lines on a cubic surface can be developed to straight lines on these "hypersurfaces" of higher degrees. Wolfson used this method to find new, simpler formulas for polynomials of certain degrees. It turns out that even if you fail to imagine a polynomial of the 100th degree, you can find its roots by “simply” finding a plane on a multidimensional cubic hypersurface (in this case, it will have 47 dimensions).
Using this new method, Wolfson confirmed the value of the resolvent degree found by Hilbert for ninth degree polynomials. And for polynomials of some other degrees - in particular, degrees above 9 - his method narrows the range of possible values of the degree of the resolvent.
So this is not a direct attack on Hilbert's 13th problem, but an approach to polynomials in general. “They found some related questions and were able to make progress on them, hoping that this would shed light on the original question,” McMullen said. And their work indicates new ways of working with these mathematical constructs.
The general theory of the degree of the resolvent also shows that Hilbert's conjectures regarding equations of the sixth, seventh, and eighth order are equivalent to other problems known in seemingly unrelated areas of mathematics. The degree of the resolvent, according to Farb, offers a way to organize these problems in terms of algebraic complexity, rather than group them into complexity classes.
And although the theory originated from Hilbert's 13th problem, mathematicians are not sure that it can solve the open question about polynomials of the seventh degree. It touches on gigantic, unexplored mathematical scales in unimaginable dimensions, but at smaller values of the degrees it encounters insurmountable obstacles and is unable to determine the degrees of the resolvent for them.
For McMullen, the lack of progress - despite hints of progress - is interesting in itself. It follows from this that the problem contains secrets that modern mathematics is simply unable to grasp. “We were not able to tackle this fundamental problem - it means that we did not enter any dark areas,” he said.
“It will take completely new ideas to solve it,” said Reichstein, who developed his own idea for simplifying polynomials, a concept he calls “basic dimension”. "It is impossible to predict where they will come from."
But the trinity does not give up. “I'm not going to give up,” Farb said. “This task has definitely become my white whale . She makes me not stop in this web of connections, and the mathematics surrounding it. "