Hlyam Hamilton invented many games, one of which is the “travel around the world” problem along the dodecahedron. In it, the tops of the dodecahedron bore the names of famous cities, and the roads connecting them were the edges. The player had to make a journey "around the world", finding a road that passes through all the peaks exactly once.
Replacing such a complex construction with a plane graph isomorphic to the original one, we obtain a problem, which we consider further in the system of zero knowledge protocols.
Zero knowledge proof

Let there be some theorem and two sides - proving and checking. The first party needs to convince the second of the truth of this statement, without revealing any other information. Thus, it can be said that "zero knowledge" of the actual proof of the theorem is transmitted.
Very strong (but not absolutely definite) evidence that the theorem is true and that the prover knows this very proof is provided by an interactive probabilistic protocol called a zero knowledge proof.
, . : " ", . , ( ).
, , , 0 . , - - , .
- ,
,
. G, .
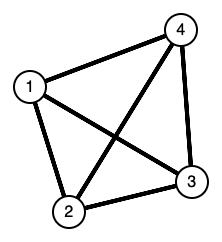
,
- , :
, .
.
, . , , , .
, .


:
, . :
,
.
C , .
, , :

1 .
, , , G, , . , , , .
, , " ".
, .
- ?
, , , "" .
, , .
, , , , G , – – .
, , , , , G , , .
.
. , , , . , , " ".
, , . , . , , .
:
Manuel Blum "How to Prove a Theorem So No One Else Can Claim It"
Schneier B. Applied Cryptography, 2nd Edition: Protocols, Algorithms, Source Texts in C // Edited by PV Semyanov. M., Triumph. - 2002.