
In our universe, the reflection of the left hand appears to be the right hand. Most of the laws of nature are symmetrical about mirror images and obey the same laws - with the exception of weak interactions. For some reason, only left-handed particles interact weakly, but not right-handed ones.
Wave your hand in the mirror and your reflection waves back at you. However, it will do it with the opposite hand from the one you are using. For most of us, this is not a problem - we can wave the other hand, and the reflection, in turn, will wave the opposite. But for the Universe, some interactions work only for left-handed particles - in particular, for particles experiencing weak interactions . No matter how we searched, we could not find their right-sided versions.
But why? Where does the Universe get such a quality, and why does it manifest itself only in the case of weak interaction? After all, strong, electromagnetic and gravitational interactions are ideally symmetrical with respect to left-side and right-side configurations. This fact in science has been verified in many experiments, and new experiments are already being prepared to verify it even more deeply. And although it is well described by the physics of the Standard Model, no one really knows why the universe works this way. Here's what we know so far.
Overcoming the quantum barrier is called the tunneling effect . This is one of the strange properties of quantum mechanics. The quantum particles themselves also have inherent properties - mass, charge, spin - which do not change after measurements.
Imagine yourself as a particle. You are moving in space, you have certain quantum properties, like mass and charge. And you also have not only the angular momentum with respect to all particles (and antiparticles) around you, but also your internal angular momentum with respect to the direction of your motion - spin . Your properties, like particles, completely determine what kind of particle you are.
With your hands, you can imagine two versions of yourself - left-handed and right-handed. First, point both of your thumbs to one side - either side, but one. Squeeze the rest of your fingers. If you now look at the thumbs so that they are directed towards you, you will see how the spins are different - all left-handed particles from this point of view "spin" clockwise [spin is directed against the movement], and right-handed ones counterclockwise [spin is directed by motion].

Left-handed polarization is inherent in 50% of the photons, and right-handed polarization is inherent in the other 50%. When a pair of particles (or a pair of particle-antiparticle) is created, their spins (their internal angular momentum) are always summed up while maintaining the total angular momentum of the system. There is nothing you can do to change the polarization of a massless particle like a photon.
Most of the time, physicists don't care about your spin - all laws and rules remain the same. The top obeys the same laws of physics, regardless of whether it is spinning clockwise or counterclockwise. The planet obeys the same rules, it revolves around an axis along or against the direction of movement in orbit. A "spinning" electron passing to the lower energy level in an atom will emit a photon regardless of the direction of its spin. In almost any circumstance, the laws of physics are said to be left-right symmetrical .
"Mirror symmetry" is one of three fundamental classes of symmetry that can be applied to particles and the laws of physics. In the first half of the 20th century, we believed that there were always conserved symmetries, three of which were:
- The symmetry of spatial parity (P), according to which the laws of physics are the same for particles and for their mirror reflections.
- Charge symmetry ©, according to which the laws of physics are the same for particles and antiparticles.
- Symmetry with respect to time reversal (T), according to which the laws of physics do not change depending on whether the system is moving forward or backward in time.
According to all the classical laws of physics, as well as general relativity and even quantum electrodynamics, these symmetries are always preserved.
Nature is not symmetric for particles / antiparticles, for specular reflections of particles, or all of these properties at once. Before the discovery of mirror symmetry breaking neutrinos, only weakly interacting particles were potential P symmetry breakers.
But to make sure that the universe is really symmetric for all these transformations, you have to test them in every possible way. The first hint that something was wrong with this picture came in 1956, when we first experimentally detected neutrinos. This particle was introduced back in 1930 by Wolfgang Pauli in the form of a tiny, neutral quantum capable of carrying away energy during radioactive decay. After such an announcement, the often quoted Pauli complained: “I have done something terrible. I postulated the existence of a particle that cannot be detected. "
Since it was predicted that neutrinos interact with ordinary matter, the cross section is negligible, Pauli did not see any realistic ways to detect them. However, after a few decades, scientists were not only able to split the atom - nuclear reactors became commonplace. According to Pauli's assumption, these reactors should produce large quantities of neutrino antiparticles - antineutrinos. A detector was built near the nuclear reactor, and the first antineutrino was discovered in 1956, 26 years later.

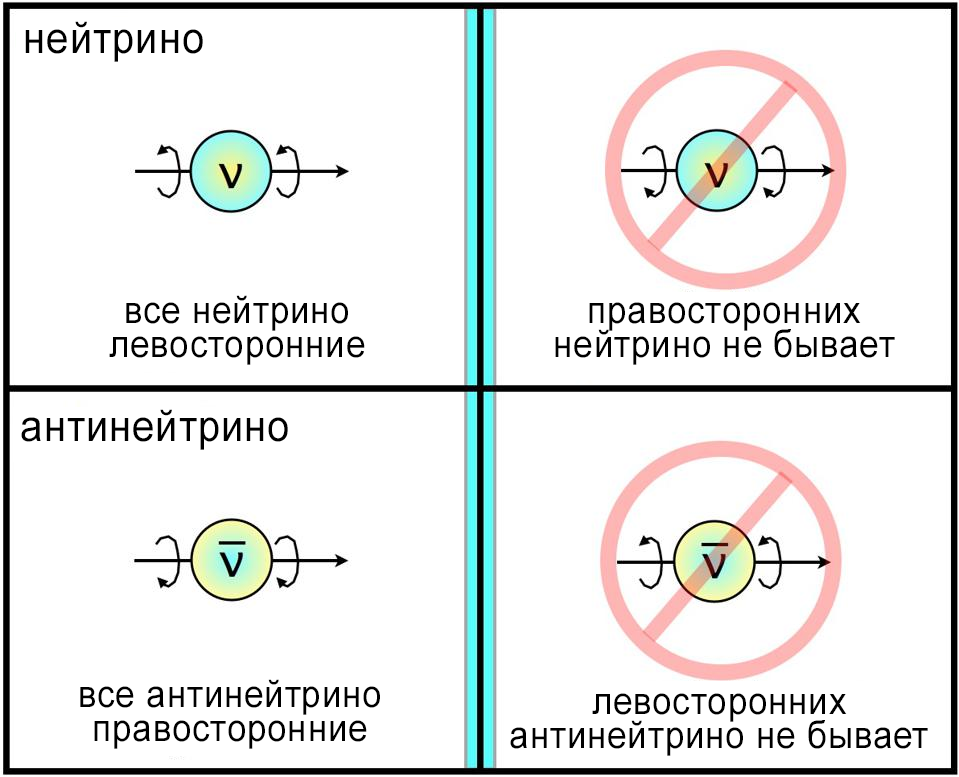
Frederick Reines, left, and Clyde Cowan, right, at the controls of the Savannah River experiment, where the electronic antineutrino was discovered in 1956. All antineutrinos are right-handed, and all neutrinos are left-handed, with no exceptions. While the Standard Model accurately describes all of this, there is no fundamental reason for it.
However, something interesting was noticed about these neutrinos: they, without exception, were right-handed, their spin was directed along their motion. Later we began to find antineutrinos as well, and found that they were all left-handed, with backward spin.
It may seem that such measurements are impossible to make. If neutrinos (and antineutrinos) are so difficult to detect because they very rarely interact with other particles, how can we even measure their spins?
The fact is that we learn their spin not as a result of direct measurements, but as a result of studying the properties of particles that appear after interaction. This is what we do with all particles that we cannot measure directly, including the Higgs boson, the only spin-zero fundamental particle known today.
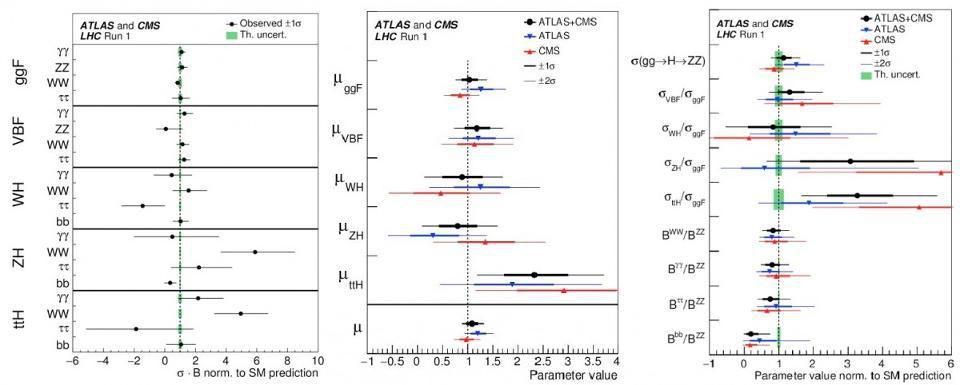
Higgs boson decay channels - observed and predicted by the Standard Model. Includes latest data from ATLAS and CMS experiments. Incredible coincidence, but also disappointing. By the 2030s, the LHC will have accumulated about 50 times more data, but the accuracy in many decay channels will still remain at the level of a few percent. The new collider could increase the accuracy by many orders of magnitude, and possibly discover the existence of new particles.
How it's done?
The Higgs boson sometimes decays into two photons, whose spin can be +1 or -1. It follows that the spin of the Higgs boson can be 0 or 2, since this will be the sum or difference of the spins of the photons. On the other hand, sometimes the Higgs boson decays into a quark / antiquark pair, each of which has a spin of + ½ or –½. Adding them up and subtracting them gives 0 or 1. One of those measurements would not give us the spin of the Higgs boson, but together they leave only one possible value, 0.
Similar technologies have been used to measure the spin of neutrinos and antineutrinos, and it comes as a surprise to most scientists that the universe and its specular reflection are not the same. If you put a mirror in front of a left-handed neutrino, its reflection will be right-handed - as in the case of the left hand, which appears to be right in the mirror. However, in our Universe there are no right-handed neutrinos, just as there are no left-handed antineutrinos. For some reason, the universe cares.

If you catch a neutrino or antineutrino moving in a certain direction, you will see that their internal angular momentum rotates either clockwise or counterclockwise, depending on whether it is a neutrino or an antineutrino.
How to comprehend all this?
Theorists Li Zhengdao and Yang Zhenning came up with the idea of parity laws, and showed that although parity seems to be a perfect symmetry, preserved in strong and electromagnetic interactions, it has not been properly tested in weak ones. Weak interactions occur when, during decay, one particle turns into another - a muon turns into an electron, a strange quark into an upward one, a neutron into a proton (when one of its downward quarks decays, turning into an upward one).
If parity was preserved, then weak interactions (all and each) would go the same for left-sided and right-sided particles. But if violated, weak interactions would occur only with left-handed particles. If only it was possible to verify this experimentally ...

Wu Jianxiong, on the left, is a remarkable and outstanding experimental physicist. She made many important discoveries that confirmed (or refuted) several important theoretical predictions. She was never awarded the Nobel Prize.
In 1956, Wu Jianxiong took a sample of cobalt-60, a radioactive isotope of cobalt, and cooled it to near absolute zero. It is known that cobalt-60 is converted into nickel-60 during beta decay. A weak interaction converts one of the neutrons in the nucleus into a proton, during which an electron and antineutrino are emitted. By applying a magnetic field to cobalt, the spins of all atoms can be aligned.
If parity were conserved, it would be possible to observe both the emitted electrons - also known as beta particles - would have both parallel and antiparallel spins. If parity was violated, then all the emitted electrons would be antiparallel. The tremendous result of Wu's experiment was not only that all the emitted electrons were antiparallel, but that they were as antiparallel as possible theoretically. A few months later Pauli wrote in a letter to Victor Weisskopf : "I can't believe that God is a weak left-hander."

, – , . , . – «». .
However, only left-handed particles are involved in the weak interaction - at least judging by our measurements. In this regard, an interesting question arises, on which we have not yet carried out measurements: when photons participate in weak interaction, do both left-handed and right-handed photons play a role in it, or only left-handed ones? For example, an adorable quark (b) turns into a strange one (s) in weak interactions, which usually happens without the participation of photons. However, a tiny fraction of b quarks, less than 1 in a thousand, will still turn into an s quark with the emission of a photon. The phenomenon is rare, but you can study it.
It is expected that such a photon should always be left-handed. We believe that parity in the Standard Model works like this (breaking in weak interactions). But if the photon can sometimes turn out to be right-handed, another crack will appear in our current understanding of physics. Among the predictions of the results of such a decay are the following:
- unexpected polarization of a photon,
- the percentage of different cases of decay that is different from the expected,
- violation of CP-invariance .
Best of all, such opportunities can be studied by the LHCb collaboration at CERN. Recently, they just set the most stringent limit ever on the possibility of right-handed photons. If the graph below, as a result of further experiments, bends so that it ceases to include the origin of coordinates (0, 0), this will mean that we have discovered a new physics.

The real and imaginary parts of the right-handed (C7-prime) and left-handed (C7) Wilson coefficients in particle physics must remain around the point (0, 0) for the Standard Model to remain correct. Measurements of various decays involving b-quarks and photons help to impose the strictest restrictions on these conditions. In the near future, the LHCb collaboration threatens to carry out even more accurate measurements.
We can definitely say that the Universe is ideally symmetric with respect to mirror images, the replacement of particles by antiparticles, the direction of time in which processes unfold - for all interactions and forces, except one. In weak interactions, and only in them, these symmetries are not preserved. All measurements that we have taken show that Pauli would have remained at a loss today. 60 years after the first discovery of symmetry breaking, it appears that weak interactions are associated only with left-handed particles.
Since neutrinos have mass, one of the most amazing experiments would be one in which we could get very close to the speed of light. Then we would overtake the left-handed neutrino so that its spin from our point of view would change to the opposite one. Would a particle suddenly show the properties of a right-handed antineutrino? Or would it become right-handed but still behave like a neutrino? Whatever characteristics it has, it could reveal to us new information about the fundamental nature of the Universe. Until that day, our best opportunity to figure out if the universe is really as left-handed as it seems to us would be indirect measurements. Just such an experiment is now underway at CERN, where they are looking for a double neutrinoless beta decay .